discrete differential geometry
My research lies in the area of discrete differential geometry. You can find an introduction in my lecture notes I gave at Kyushu University, Japan in 2008.
recent preprints
- Tim Hoffmann, Jannik Steinmeier, and Gudrun Szewieczek. Discrete curve theory in space forms: planar elastic and area-constrained elastic curves, 2025 [ bib | arXiv ]
- Alexander I. Bobenko, Tim Hoffmann, and Nina Smeenk Constant mean curvature surfaces from ring patterns: Geometry from combinatorics, 2024. [ bib | arXiv ]
- Tim Hoffmann and Gudrun Szewieczek. Isothermic nets with spherical parameter lines from discrete holomorphic maps, 2024. [ bib | arXiv ]
mathematical visualization
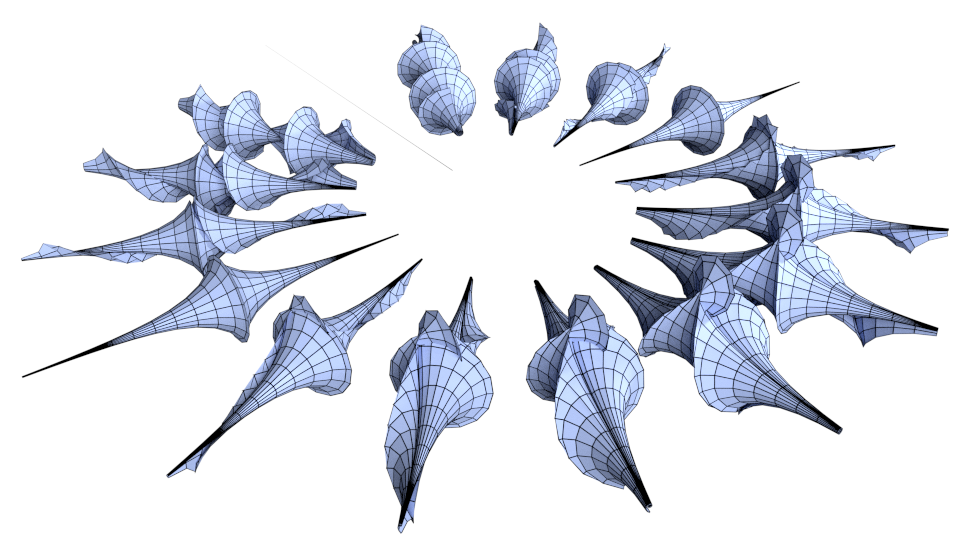
An isothermic torus with one family of planar and one family of spherical curvature lines that generates a compact Bonnet pair (Bonnet pairs are non congruent pairs of surfaces that are isometric and have the same mean curvature). We could prove the existence of Bonnet tori here.
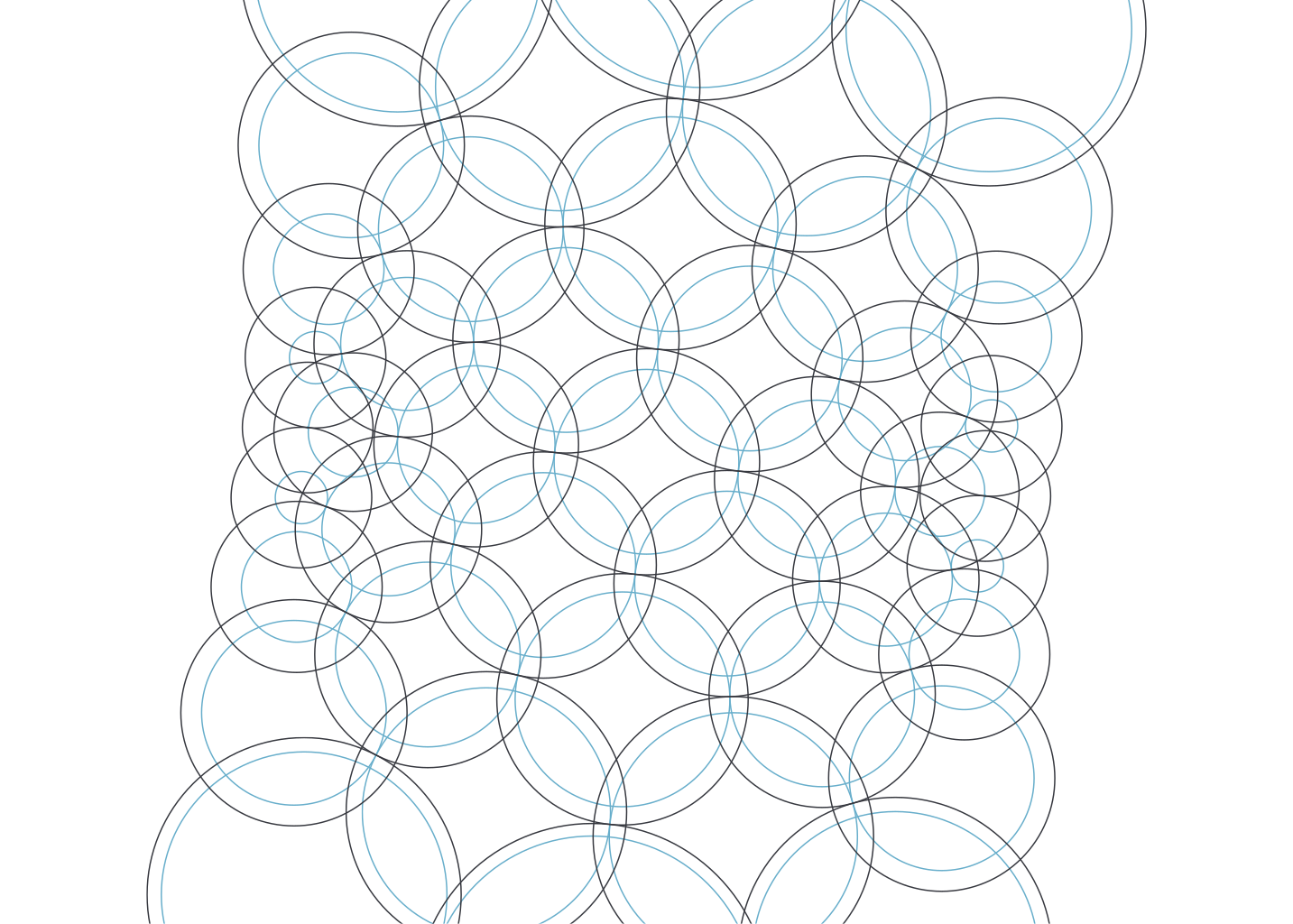
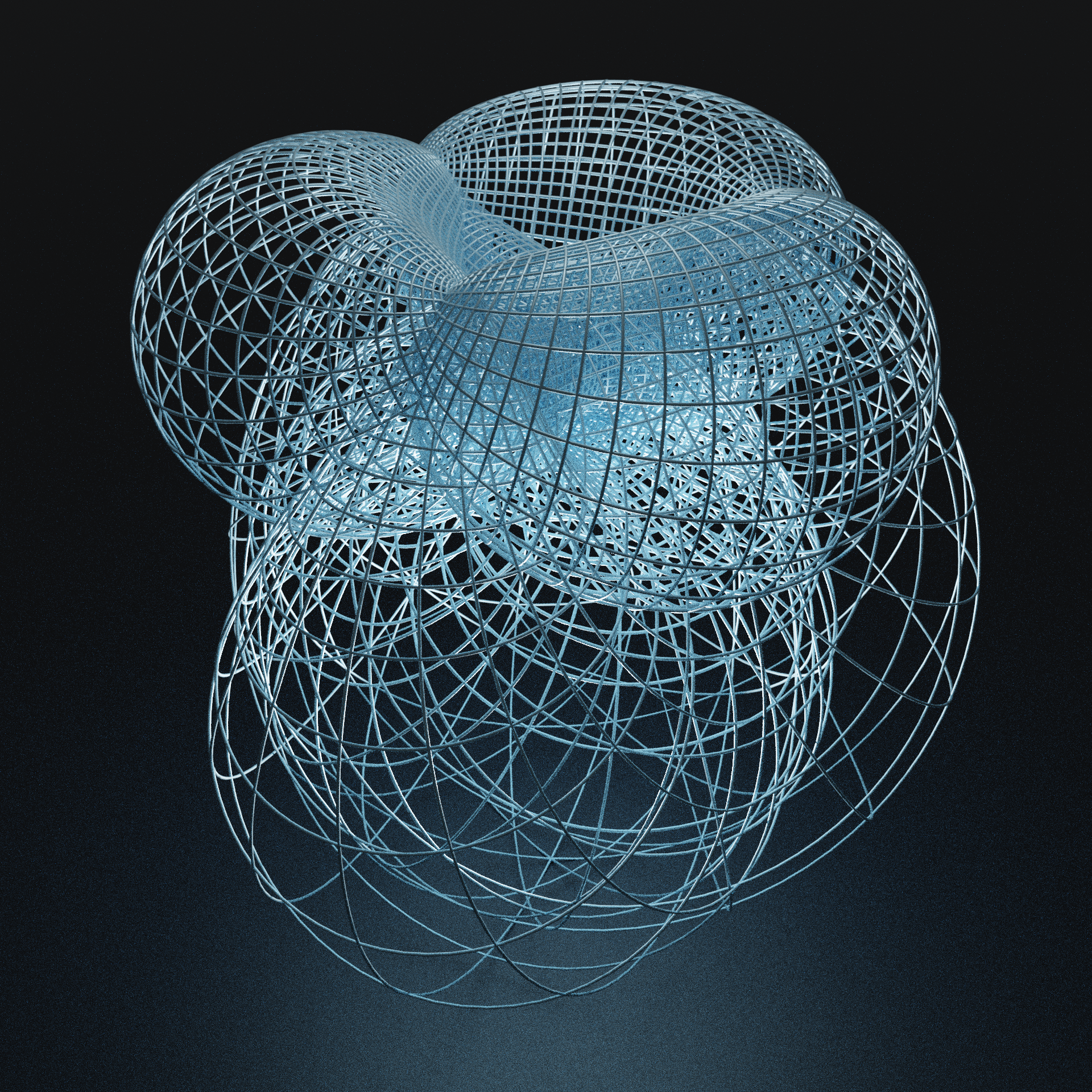
An Erf ring pattern. Orthogonal Ring patterns are a generalization of orthogonal circle patterns: Each ring consists of a pair of concentric circles and for each lattice direction one of them intersects its corresponding neighbour orthogonally. The theory behind them is written here.

Discrete orthogonal geodesic nets (DOG nets for short) are a discretization of developable surfaces, that utilizes the fact that a surface is developpable if and only if it admits an orthogonal net of geodesics. The advantage of such a parametrization by orthogonal geodesics — compared to one by rulings for example — is, that it is invariant under isometric deformations. One can read about them here and here.
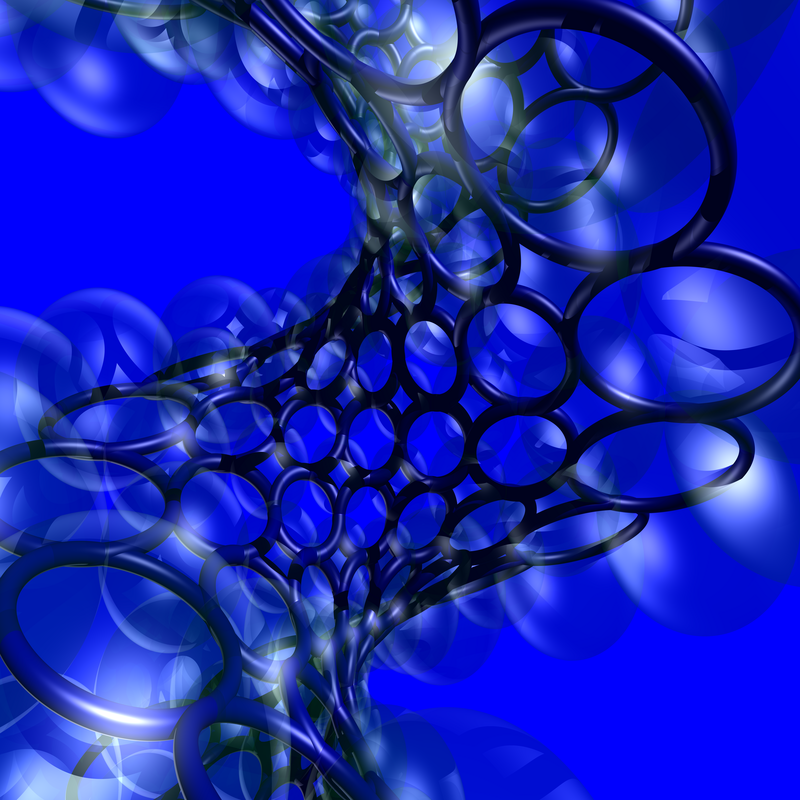
A surface in the associated family of an s-isothermic Enneper surface. S-isothermic nets are made from orthogonal circle patterns (although the associated family of them is no longer orthogonal). One can read about them here.
A 3d printed circle pattern on the sphere, that stereographically projects to the regular pattern in the plane. This can be seen as the shadow of the circles under a point light at the northpole is exactly the stereographic projection.